Organic Chemistry Text Book (CHEM 3401 and 3402)
5.4 Optical Activity, Symmetry, and Chirality
As chemists studied organic compounds isolated from plants and animals, a new and subtle type of configurational stereoisomerism was discovered. For example, lactic acid ( a C3H6O3 carboxylic acid) was found in sour milk as well as in the blood and muscle fluids of animals. The physical properties of this simple compound were identical, regardless of the source (m.p, 53 ºC & pKa 3.80), but there was evidence that the physiological behavior of the compound from the two sources was not the same. Another natural product, the fragrant C10H14O ketone carvone, was isolated from both spearmint and caraway. Again, all the physical properties of carvone from these two sources seemed to be identical (b.p. 230 ºC), but the odors of the two carvones were different and reflected their source. Other examples of this kind were encountered, and suspicions of a subtle kind of stereoisomerism were confirmed by the different interaction these compounds displayed with plane polarized light. We now know that this configurational stereoisomerism is due to different right and left-handed forms that certain structures may adopt, in much the same way that a screw may have right or left-handed threads but the same overall size and shape. Isomeric pairs of this kind are termed enantiomers (from the Greek enantion meaning opposite).
Chirality |
|---|
Chirality and Symmetry
All objects may be classified with respect to a property we call chirality (from the Greek cheir meaning hand). A chiral object is not identical in all respects (i.e. superimposable) with its mirror image. An achiral object is identical with (superimposable on) its mirror image. Chiral objects have a "handedness", for example, golf clubs, scissors, shoes and a corkscrew. Thus, one can buy right or left-handed golf clubs and scissors. Likewise, gloves and shoes come in pairs, a right and a left. Achiral objects do not have a handedness, for example, a baseball bat (no writing or logos on it), a plain round ball, a pencil, a T-shirt and a nail. The chirality of an object is related to its symmetry, and to this end it is useful to recognize certain symmetry elements that may be associated with a given object. A symmetry element is a plane, a line or a point in or through an object, about which a rotation or reflection leaves the object in an orientation indistinguishable from the original. Some examples of symmetry elements are shown below.
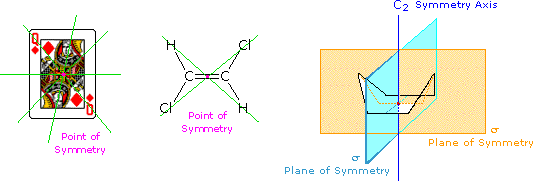
The face playing card provides an example of a center or point of symmetry. Starting from such a point, a line drawn in any direction encounters the same structural features as the opposite (180º) line. Four random lines of this kind are shown in green. An example of a molecular configuration having a point of symmetry is (E)-1,2-dichloroethene. Another way of describing a point of symmetry is to note that any point in the object is reproduced by reflection through the center onto the other side. In these two cases the point of symmetry is colored magenta.
The boat conformation of cyclohexane shows an axis of symmetry (labeled C2 here) and two intersecting planes of symmetry (labeled σ). The notation for a symmetry axis is Cn, where n is an integer chosen so that rotation about the axis by 360/nº returns the object to a position indistinguishable from where it started. In this case the rotation is by 180º, so n=2. A plane of symmetry divides the object in such a way that the points on one side of the plane are equivalent to the points on the other side by reflection through the plane. In addition to the point of symmetry noted earlier, (E)-1,2-dichloroethene also has a plane of symmetry (the plane defined by the six atoms), and a C2 axis, passing through the center perpendicular to the plane. The existence of a reflective symmetry element (a point or plane of symmetry) is sufficient to assure that the object having that element is achiral. Chiral objects, therefore, do not have any reflective symmetry elements, but may have rotational symmetry axes, since these elements do not require reflection to operate. In addition to the chiral vs achiral distinction, there are two other terms often used to refer to the symmetry of an object. These are:
(i) Dissymmetry: The absence of reflective symmetry elements. All dissymmetric objects are chiral.
(ii) Asymmetry: The absence of all symmetry elements. All asymmetric objects are chiral.
Models of some additional three-dimensional examples are provided on the interactive symmetry page.
|
The symmetry elements of a structure provide insight concerning the structural |
Optical Activity
Identifying and distinguishing enantiomers is inherently difficult, since their physical and chemical properties are largely identical. Fortunately, a nearly two hundred year old discovery by the French physicist Jean-Baptiste Biot has made this task much easier. This discovery disclosed that the right- and left-handed enantiomers of a chiral compound perturb plane-polarized light in opposite ways. This perturbation is unique to chiral molecules, and has been termed optical activity.
Plane-polarized light is created by passing ordinary light through a polarizing device, which may be as simple as a lens taken from polarizing sun-glasses. Such devices transmit selectively only that component of a light beam having electrical and magnetic field vectors oscillating in a single plane. The plane of polarization can be determined by an instrument called a polarimeter, shown in the diagram below.
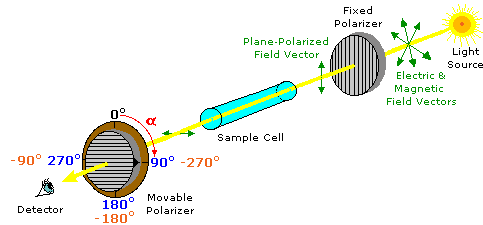
Monochromatic (single wavelength) light, is polarized by a fixed polarizer next to the light source. A sample cell holder is located in line with the light beam, followed by a movable polarizer (the analyzer) and an eyepiece through which the light intensity can be observed. In modern instruments an electronic light detector takes the place of the human eye. In the absence of a sample, the light intensity at the detector is at a maximum when the second (movable) polarizer is set parallel to the first polarizer (α = 0º). If the analyzer is turned 90º to the plane of initial polarization, all the light will be blocked from reaching the detector.
András Szilágyi has created a nice animation, illustrating various kinds of polarized light. This site may be examined by Clicking Here .
Chemists use polarimeters to investigate the influence of compounds (in the sample cell) on plane polarized light. Samples composed only of achiral molecules (e.g. water or hexane), have no effect on the polarized light beam. However, if a single enantiomer is examined (all sample molecules being right-handed, or all being left-handed), the plane of polarization is rotated in either a clockwise (positive) or counter-clockwise (negative) direction, and the analyzer must be turned an appropriate matching angle, α, if full light intensity is to reach the detector. In the above illustration, the sample has rotated the polarization plane clockwise by +90º, and the analyzer has been turned this amount to permit maximum light transmission.
The observed rotations (α) of enantiomers are opposite in direction. One enantiomer will rotate polarized light in a clockwise direction, termed dextrorotatory or (+), and its mirror-image partner in a counter-clockwise manner, termed levorotatory or (–). The prefixes dextro and levo come from the Latin dexter, meaning right, and laevus, for left, and are abbreviated d and l respectively. If equal quantities of each enantiomer are examined , using the same sample cell, then the magnitude of the rotations will be the same, with one being positive and the other negative. To be absolutely certain whether an observed rotation is positive or negative it is often necessary to make a second measurement using a different amount or concentration of the sample. In the above illustration, for example, α might be –90º or +270º rather than +90º. If the sample concentration is reduced by 10%, then the positive rotation would change to +81º (or +243º) while the negative rotation would change to –81º, and the correct α would be identified unambiguously.
Since it is not always possible to obtain or use samples of exactly the same size, the observed rotation is usually corrected to compensate for variations in sample quantity and cell length. Thus it is common practice to convert the observed rotation, α, to a specific rotation, [α], by the following formula:
| Specific Rotation = |  |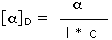
where l = cell length in dm, c = concentration in g/ml | |
| D is the 589 nm light from a sodium lamp |
Compounds that rotate the plane of polarized light are termed optically active. Each enantiomer of a stereoisomeric pair is optically active and has an equal but opposite-in-sign specific rotation. Specific rotations are useful in that they are experimentally determined constants that characterize and identify pure enantiomers. For example, the lactic acid and carvone enantiomers discussed earlier have the following specific rotations.
| Carvone from caraway: [α]D = +62.5º | this isomer may be referred to as (+)-carvone or d-carvone | |
| Carvone from spearmint: [α]D = –62.5º | this isomer may be referred to as (–)-carvone or l-carvone | |
| Lactic acid from muscle tissue: [α]D = +2.5º | this isomer may be referred to as (+)-lactic acid or d-lactic acid | |
| Lactic acid from sour milk: [α]D = –2.5º | this isomer may be referred to as (–)-lactic acid or l-lactic acid |
A 50:50 mixture of enantiomers has no observable optical activity. Such mixtures are called racemates or racemic modifications, and are designated (±). When chiral compounds are created from achiral compounds, the products are racemic unless a single enantiomer of a chiral co-reactant or catalyst is involved in the reaction. The addition of HBr to either cis- or trans-2-butene is an example of racemic product formation (the chiral center is colored red in the following equation).
| CH3CH=CHCH3 + HBr |  |
(±) CH3CH2CHBrCH3 |
Chiral organic compounds isolated from living organisms are usually optically active, indicating that one of the enantiomers predominates (often it is the only isomer present). This is a result of the action of chiral catalysts we call enzymes, and reflects the inherently chiral nature of life itself. Chiral synthetic compounds, on the other hand, are commonly racemates, unless they have been prepared from enantiomerically pure starting materials.
There are two ways in which the condition of a chiral substance may be changed:
1. A racemate may be separated into its component enantiomers. This process is called resolution.
2. A pure enantiomer may be transformed into its racemate. This process is called racemization.